Once it is found that either wanted or unwanted prism is present in any given lens and the direction of the base is determined, the power of the prism can then be calculated utilizing a simple formula called Prentice’s Rule. It is important to become familiar with Prentice’s Rule since it may be used as a routine matter in the optical dispensary.
Prentice’s Rule: Calculating Prismatic Effect
Prentice’s Rule states:
The power of the prism is equal to the power of the lens in diopters times the amount of decentration in millimeters divided by 10.
Stated Algebraically:
Δ = (F x dec (mm)) / 10
where:
Δ = prism diopters
F = power of the lens in diopters
dec = decentration or distance in mm away from the optical center of the lens.
By applying this formula, opticians can determine the amount of prism experienced by the patient and the direction of the prism’s base, which is crucial in understanding and addressing visual imbalances.
Prism Direction
Prism does not just affect focal length and power. Where it is positioned in the lens also matters. Prism, as written in a prescription, is measured in diopters and further defined by the direction of the prism base. Prescribers use the following abbreviations most often:
- BU: Base up
- BD: Base down
- BI: Base in
- BO: Base out
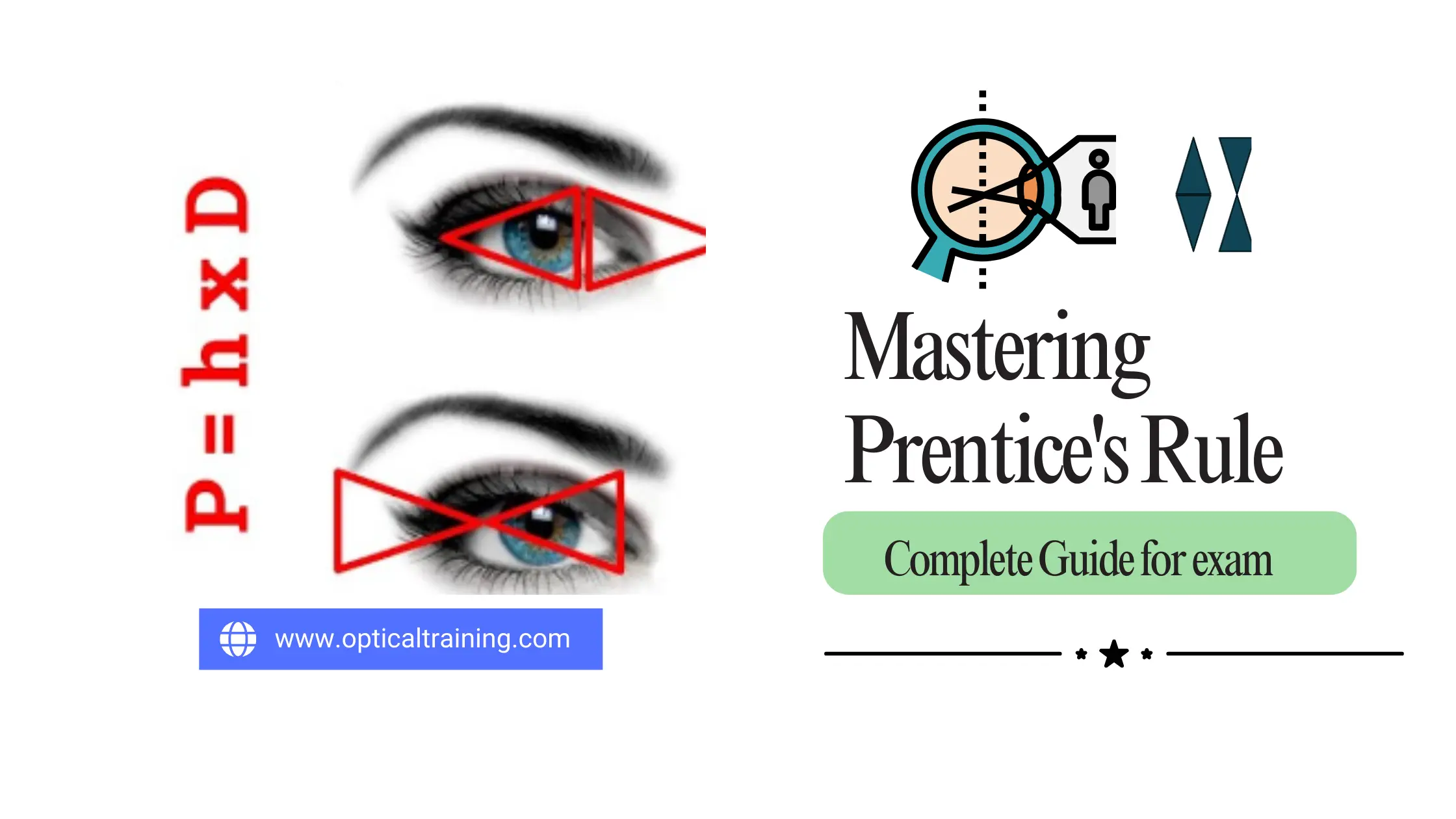
The illustration below visually illustrates the prism directions in minus lenses.

The direction of the prism base depends on the OC positioning relative to the pupil, as well as the type of lens being used. An easy way to remember the direction of the base for a minus lens, is to remember that the base direction will always be in the opposite direction of the decentration. As illustrated in Figure D above, when the OCs are inward of the PDs, the result is Base Out prism. Drawing triangles that represent the OC as where the apexes meet is a great visual tool.
The illustration below visually illustrates the prism directions in minus lenses.

An easy way to remember the direction of the base for a plus lens, is to remember that the base direction will always be in the same direction of the decentration. As illustrated in Figure D above, when the OCs are inward of the PDs, the result is Base In prism.
Adding and Canceling Prism
The induced prism in the right lens might add to or offset the induced prism in the left lens. We call these two functions adding and canceling prism.
Canceling Prism
Canceling prism bases means that the prism in the right lens will cancel out all or part of the prism in the left lens. The canceling base directions are listed below:
- Up/Up
- Down/Down
- In/Out
- Out/In
Adding Prism
Adding prism is exactly what it sounds like. You add the amount of prism in the right lens to the amount in the left lens for a total imbalance. The adding base directions are listed below:
- Up/Down
- Down/Up
- In/In
- Out/Out
Example One:
1 ∆ prism base In OD and 1 ∆ base Out in OS cancel each other out. Subtract 1 from 1, and the total prism is 0.
Example Two:
1 ∆ base In and 1 ∆ base In are adding, added together is a total imbalance of 2 ∆.
For optician training for ABO NOCE study: Prentice’s Rule is used to determine the amount of prismatic effect created by a lens or lenses. It works by using the power of the lens, the position of the lens and the distance that the lens OC is from where it was intended to be. These examples below are good examples to study.
Prentice’s Rule Practice
Example 1:
How much prism is induced 4 mm away from the optical center of a +3.00 D spherical lens?
Applying Prentice’s Rule, F = +3.00 and dec = 4.0 mm, so:
Δ = (F x dec) / 10
= (+3.00 x 4) / 10
= 1.2 prism diopters
Example 2:
A patient’s PD was mistakenly noted to be 62 mm when the actual measurement is 66 mm. If the power of the lenses are −2.00 diopters in each eye, how much prism will be induced in the finished spectacles and in which direction is the base?
Applying Prentice’s Rule, F = -2.00 per eye and dec = 2.0 mm per eye, so:
Δ = (F x dec) / 10
Right Eye: (-2.00 x 2) / 10 = 0.4 prism diopters
Left Eye: (-2.00 x 2) / 10 = 0.4 prism diopters
Total: 0.8 prism diopters Base Out
How do we know the direction of the prism is base out?
The optical centers were positioned a total of 4 mm narrower than the actual PD. By referring to the figure D on the minus lens illustration above, it can be observed that the direction of the base of the prism through which the patient is looking is oriented out, away from the nose. Remember, minus lenses will result in a prism base direction opposite to the decentration direction. So, when the OCs are narrower than the PDs, the result is Base Out prism.
Example 3:
Patient’s Rx:
-4.00 -1.00 x 150
-5.00 -2.00 x 120
Patient’s actual PD: 31/34
Finished spectacles OC: 34/31
This example is working with toric lenses, so there will be additional steps to calculate the amount of prism. The first basic concept to understand is that when working with prism errors related to PDs, the error is along the horizontal meridian, or 180 degree meridian. Conversely, if you were calculating vertical prism, the error would be along the vertical meridian, or 090 degree meridian. Therefore, in order to calculate the horizontal prismatic effect, the power in each lens needs to be at 180 degrees. If the provided patient prescription happened to be at the 090 degree meridian, you would be able to flat transpose it to be at 180 degrees. In our provided example, this is not the case so flat transposition is not possible.
The next item to introduce is the Cylinder Power in Various Meridians chart below. This is often remembered as the 30, 45, 60 Rule. By memorizing the chart below, you will know how much of the cylinder power is to be used when determining the total diopter power to plug into Prentice’s Rule.
| Degrees Away from Cylinder | % of Cylinder Power in Effect |
|---|---|
| 0 | 0 |
| 30 | 25 |
| 45 | 50 |
| 60 | 75 |
| 90 | 100 |
So, you need to determine how much cylinder power is going to be used in the Prentice’s Rule formula. You’ll first need to determine how many degrees each of the axes are away from 180 degrees.
The right lens axis is 150 degrees.
180 – 150 = 030 degrees away
The left lens axis is 120 degrees.
180 – 120 = 060 degrees away
Now you can use the 30, 45, 60 Rule chart to determine how much of the cylinder power to use.
The right lens axis is 030 degrees away, and therefore, 25% of the cylinder power will be used. To calculate, simply times the cylinder power (-1.00) by the percentage in decimal form (0.25).
-1.00 x 0.25 = -0.25
The left lens axis is 060 degrees away, and therefore, 75% of the cylinder power will be used. To calculate, times the cylinder power (-2.00) by the percentage in decimal form (0.75).
-2.00 x 0.75 = -1.50
Now, you will add the calculated cylinder powers to each sphere power to determine the total diopter power to be used in the formula.
The right lens sphere power (-4.00) + the calculated cyl power (-0.25) = -4.25
The left lens sphere power (-5.00) + the calculated cyl power (-1.50) = -6.50
Therefore, the information you’ll need to calculate prism on this order is below:
Patient’s prescription (newly calculated diopter powers):
-4.25
-6.50
Patient’s actual PD: 31/34
Finished spectacles OC: 34/31
The decentration amount on the right lens is 3 mm. The decentration amount on the left lens is 3 mm.
You can now plug the values into the formula and solve. Be sure to round as necessary.
Δ = (F x dec) / 10
Right Eye: (-4.25 x 3) / 10 = 1.3 prism diopters BI
Left Eye: (-6.50 x 3) / 10 = 2.0 prism diopters BO
Total: 0.7 prism diopters Base Out
Consider how we calculated the prism directions for each lens, and how we determined the total prismatic effect from the two lenses. The right lens OC is wider than the PD, and is a minus lens, so therefore is Base In. The left lens OC is narrower than the PD, and is a minus lens, so therefore is Base Out. Base In and Base Out is a canceling effect, so instead of adding the prism amount together, you would subtract them. Then, you keep the direction of the larger prism amount. The result is 0.7 Base Out total prismatic effect.
Vertical Imbalance at the Reading Level
Consider a patient with the following prescription:
- Right eye: -0.25 +3.00 x 180, Add +1.50
- Left eye: -1.00 +1.00 x 90, Add +1.50
Assuming the reading level is 10 mm below the optical center, we can calculate the total power in the vertical meridian (090 degree meridian) for each lens:
Right eye: First transpose the Rx to be in the 090 degree meridian: +2.75 -3.00 x 090
Then, because we are now 0 degrees away from 090 degrees, the cylinder will be dropped: +2.75
You then combine the new sphere power with the Add power:
+ 1.50 = +4.25 D
Left eye: The axis is already on the 090 degree meridian, and therefore, is 0 degrees away. The cylinder will be dropped:
-1.00
You then combine the new sphere power with the Add power:
-1.00 + 1.50 = +0.50 D
Using Prentice’s Rule, we can determine the prismatic effect at the reading level:
Δ = (F x dec) / 10
Right Eye: (+4.25 x 10) / 10 = +4.25 D
Left Eye: (+0.50 x 10) / 10 =+0.50 D
The difference between the two eyes is 3.75 diopters of vertical imbalance, which may require corrective measures.
Prism by Decentration
Prentice’s Rule can also be used in reverse to determine the amount of decentration required to induce a specific amount of prism. The formula can be rearranged as:
Dec = (Δ / F) x 10
Where:
- Dec is the distance from the optical center in millimeters
- Δ is the desired prism in diopters
- F is the power of the lens in the meridian of the prism base
For example, if you need to induce 1.6 diopters of prism in a 4.00 D lens, the required decentration would be:
Dec = (Δ / F) x 10
Dec = (1.6 / 4.00) x 10 = 4mm
Similarly, if you know the decentration and the resulting prism, you can use the formula to determine the power of the lens being decentered.
Ready to take your optical knowledge to the next level? Stay updated with the latest industry trends, news, and updates by joining our email newsletter. Don’t miss out—subscribe today and stay ahead in your optical career!




